What about the total amount of water coming off the roof? Suppose it is raining, so insulation doesn’t make any difference. Again, about twice as much water would flow off a given spot along the eaves for our house. But the total amount of water flowing off one side of the house is determined by the total area of the roof upstream.
Consider the one-meter section of our house in Figure 2. Let’s estimate how much water would flow off a meter length on the east side for a rainfall of 1 centimeter per hour. The roof measures 10 meters from the top to the eaves.
In an hour, the volume of water falling on the roof would be 1 centimeter per hour or 0.01 meters per hour × 1 meter × 10 m, or 0.1 cubic meter of water per hour. Since water weighs 1 gram per cubic centimeter and there are 100 x 100 x 100 x 0.1 cubic centimeters in 0.1 cubic meter, about 100 kilograms of water fall on this one-meter section of the roof per hour. The same amount flows over a meter section of the eaves to the ground in about an hour (assuming the roof drains as fast as it rains!)
But we need to make a minor correction for the fact that the roof is not exactly horizontal (i.e., it’s covering less ground).
If the angle of the roof to the ground is 20 degrees, we need to multiply the 100 kilograms of water per hour per 1 meter by 0.94, making the total rainfall 94 kilograms.
If the roof measures 10 meters along the eaves and top of the roof (Figure 3), the total amount of water flowing off the roof on the east side is 10 times that amount, or 940 kilograms allowing for the angle of the roof.
What about the roof of the imaginary house in Figure 3, which measures 5 meters from the top of the roof to the eaves? Half as much water or 47 kilograms falls on a one-meter slide of this house (Figure 2) each hour, so half as much water will flow off the eaves per meter each hour compared to our house, which measures 10 meters from roof to eaves. We assume the roof’s angle to the ground is the same as our house.
The total amount of water flowing off the roof of the imaginary house each hour would then be:
47 kilograms per 1 meter along the eaves times 20 meters, or 940 kilograms flowing off the east side of the roof each hour. This is of course the same amount of water flowing off our house.
Did you notice that if you just know that the area and angle of the roof of the imaginary house are the same as the roof as our house, means that the total amount of rain falling on both houses is the same, and therefore the same amount of water flowing off the two roofs is the same?
We could call these roofs “roof watersheds” or “roofsheds” because they shed water – in the form of icicles in the first example, or in the form of liquid in the second.
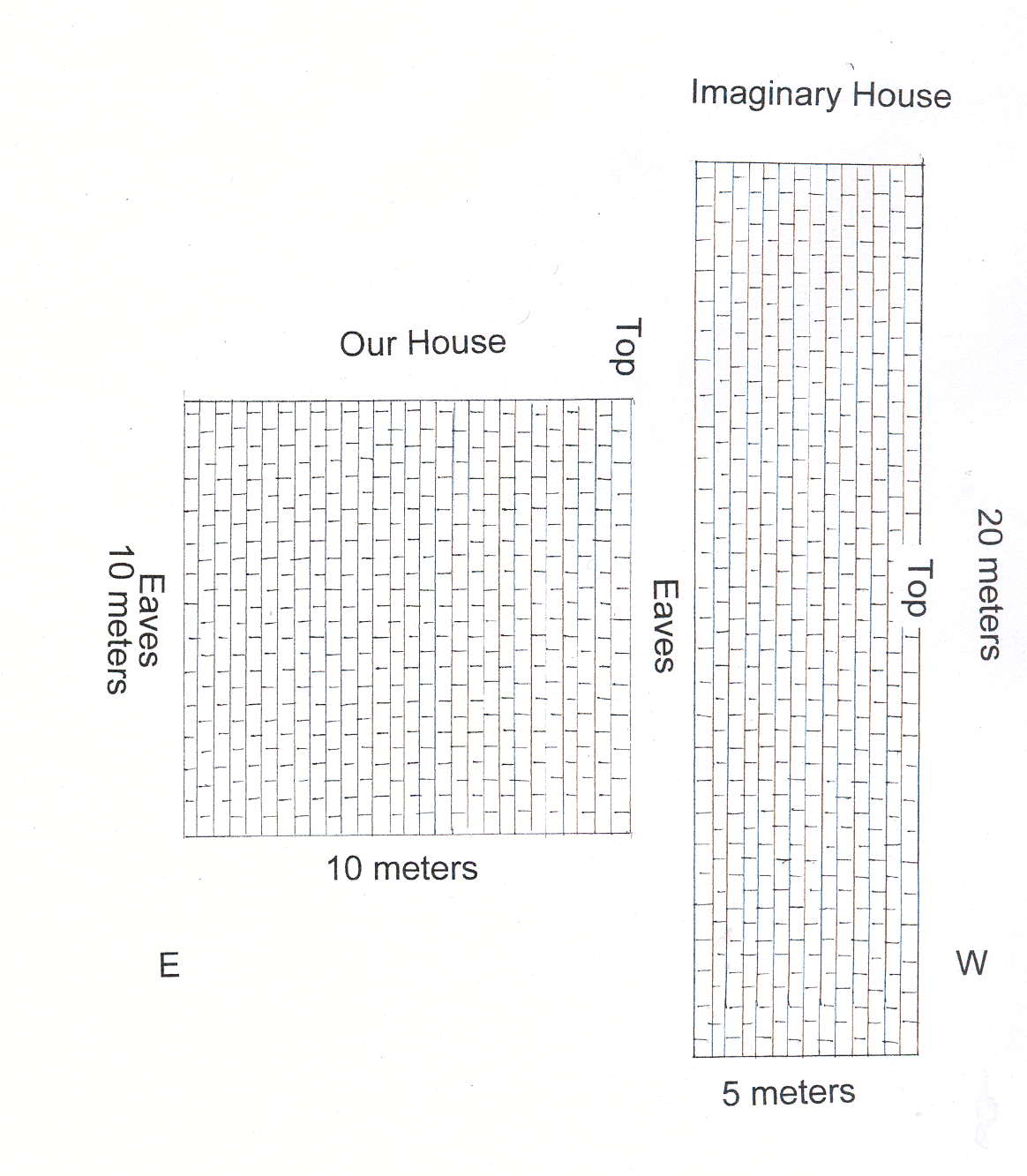
Figure 3. “Roofsheds” for our house and the imaginary house, viewed from above. Both roofs, having the same area (100 square meters) and angle to the ground (20 degrees), will shed the same amount of water on the east side, where the eaves are.

I’m just curious, wouldn’t the pitch of the roof also impact the rate at which the water runs off? For example, using the roof sheds in your Fig. 3 and assuming a constant height for the attic crawl space, say 2m, the angle of your roof would be much less than for the imaginary roof. As a result, I would expect the water to flow off it more slowly. This would also affect the icicles you mentioned in a prior blog. If your home has a shallow pitch compared to your neighbors, would not more of it be apt to freeze forming longer and wider icicles?
The major effect of the pitch of the roof is affecting how much water falls on it. So a very shallow roof would have more rain on it for the same area.
Suppose you had two roofs with equal “footprints” so that they received the same amount of rain. In steady state the flow off the roofs would be equal.
But if you rolled a marble off the longer, steeper roof, it would certainly be going faster.
I suppose the key is that the roofs would have the same flow off of them for steady state, but not for an “impulse”.